In the second test we compare three-dimensional models of strongly magnetized supersonic turbulence in both cases: classical MHD with isotropic pressure, and kinetic MHD with anisotropic pressure.The Alfven and sonic Mach numbers for these models are ![]() and
and ![]() , respectively.
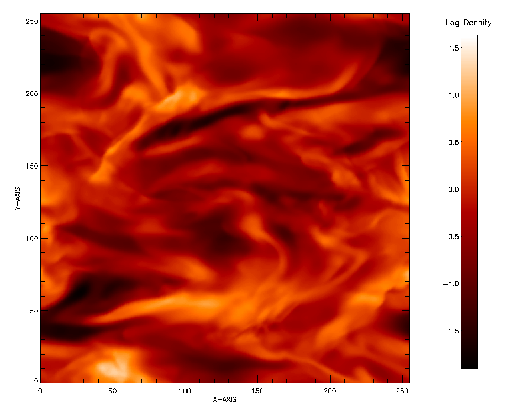
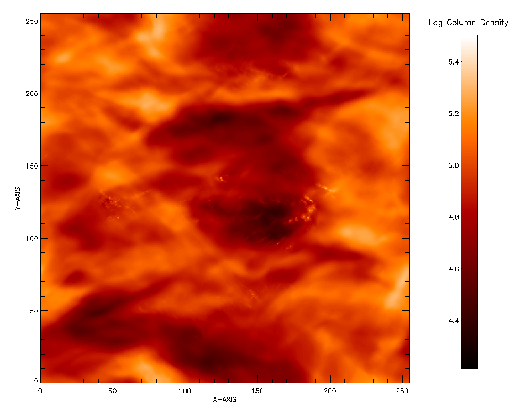
In Figure 2 we show density and column density structures developed in magnetized supersonic turbulence when the pressure is isotropic (MHD formulation) and anisotropic (CGL-MHD formulation). The density structures evolve in a very different way in both models. While the MHD turbulence produces dominating small-scale structure, the CGL-MHD model gives structures at larger scales. At the same times, the contrast of density in models with anisotropic pressure is reduces. The different type of structure generated during the turbulence evolution is visible in the maps of column densities, as well.
, respectively.
In Figure 2 we show density and column density structures developed in magnetized supersonic turbulence when the pressure is isotropic (MHD formulation) and anisotropic (CGL-MHD formulation). The density structures evolve in a very different way in both models. While the MHD turbulence produces dominating small-scale structure, the CGL-MHD model gives structures at larger scales. At the same times, the contrast of density in models with anisotropic pressure is reduces. The different type of structure generated during the turbulence evolution is visible in the maps of column densities, as well.
In Figure 3 we present the structure of magnetic field for MHD and CGL-MHD models. The magnetic field topology do not show much deviation in the case of anisotropic pressure as compared to classical MHD models of turbulence. It is justified by two facts. Firstly, the anisotropic pressure contributes directly to the motions of the fluid through the motions equations. Secondly, the strong magnetic field is less sensitive to the changes due to the magnetic tension.
 |  |
 |  |
 |  |
 |  |
 |
 |